
We started at 4:00, with roughly 100 campers making various truncated
tetrahedron modules.

There are eight different shapes to build, spread among various student
groups.

This was all happening as people were coming and going to meetings with
their advisors.

With so many people we quickly built the core and some adjacent cells
towards one outer facet.

Then we lifted it and turned it so that outer facet was on the floor
and we could work all around it.

Once you learn the pattern, it is relatively quick to assemble.

It fills a sphere about 2 meters in diameter.

Working up to the top, we had about 8000 of the pieces in place after
four
hours of work, at 8:00.

For engineering support, we had vertical rods carrying some of the
weight.
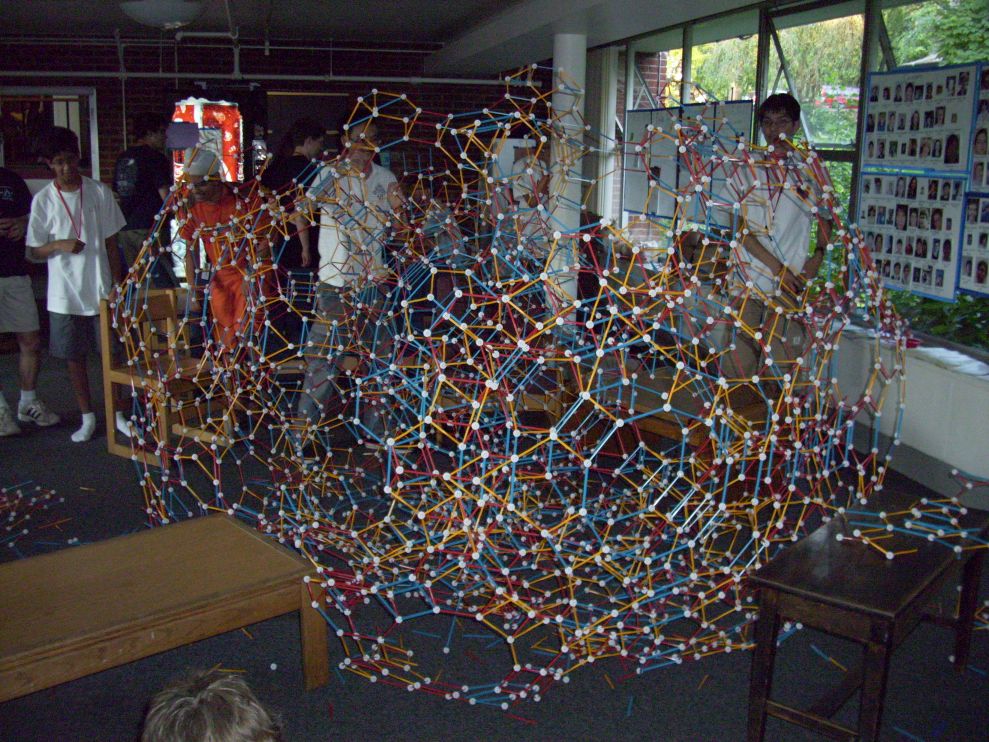
But then the bottom collapsed as we were adding more modules to the top.

Having learned a few things from the experience, we disassembled and
started again.

About 20% of the original modules could be salvaged, and we worked
faster with practice.

This time we worked more in the center at first. It clears the ceiling
by 1/4 inch.
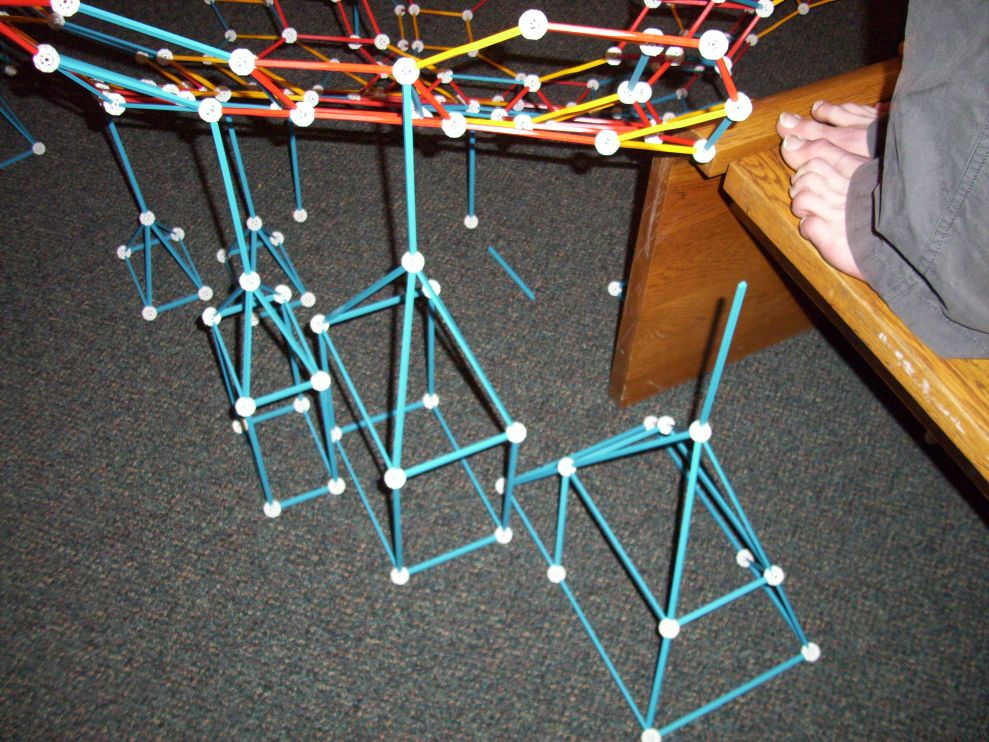
And we experimented with more and larger engineering supports for
carrying the weight.

Things went very well, with a dedicated group of hard-core Zomers.

In this structure, at each vertex there are two truncated
icosidodecahedra, a truncated tetrahedron, and a triangular prism.

A half hour after midnight, we were finished! It is my new
favorite polytope! Another name for it is the canti-truncated 120 cell.
It is the last of the fifteen H4 polytopes to be made. I have now
participated in the construction of all of them.
There are 10800 parts (plus the scaffolding). It is also the
largest ever made in terms of volume.
You can read more about these polytope models
here.

Another activity I did was a puzzle workshop, with various geometric
puzzles. These paper pieces need to be assembled.

The
H-puzzle is
made from 30 cut paper parts.

This highly interwoven
Meanders modular kirigami
construction is pretty tricky.

It looks cool when finished in a perfect five-color pattern based on
the compound of five tetrahedra..

Another activity was constructing this sculpture from 200 CDs.
It is based on a truncation of the rhombic triacontahedron.

I always do some Zometool workshops at MathCamp. Here, we are
making some polyhedral models.

And this is a 4D polytope workshop. How often do you see four
120-cells in the same room?
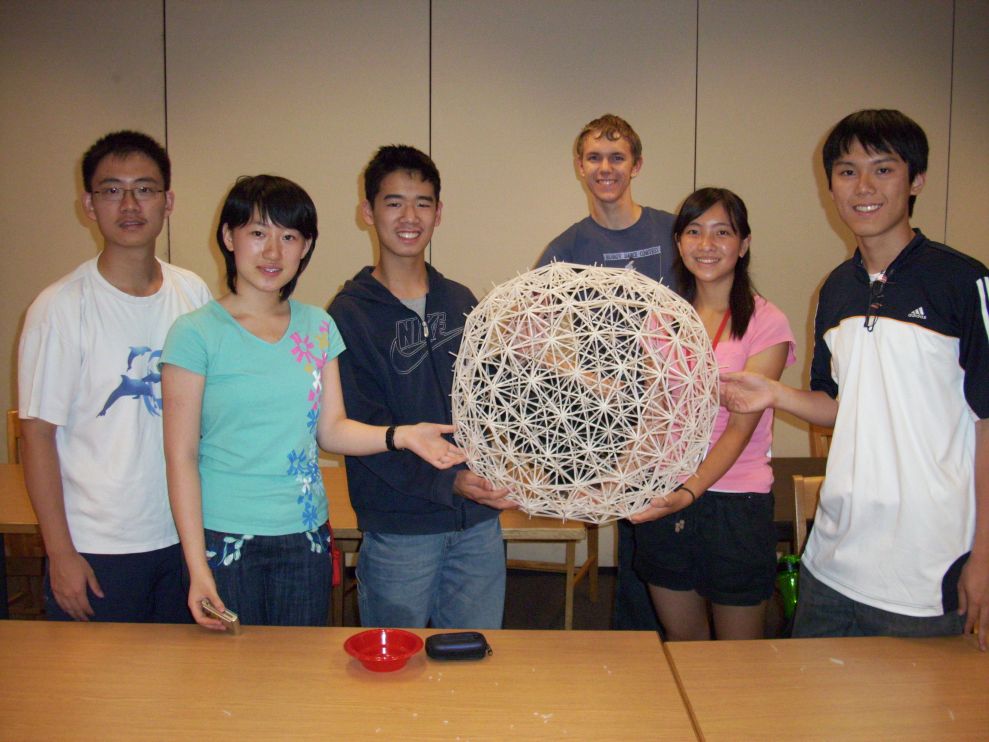
In another workshop, we made a 2-layer geodesic sphere from
TubeSpace
parts.
This is modeled after the Montreal Expo67 dome, with triangles on the
outer layer
and a dual layer of hexagons inside.
I wrote
a Mathematica program to
calculate
all the strut lengths. More information about the structure and
construction is
here.

I also took a break from math one day for a strenuous climb up Mt. St.
Helens.
This is a self-portrait at the rim of the volcano, looking way down
into the crater.
If you look carefully, you can see the steam venting out of the
central lava dome.