After you've mastered
cutting
your
bagel
into
two
linked
halves, you may want to try the knotted
cuts shown on this page. They show two ways to cut a bagel
into a
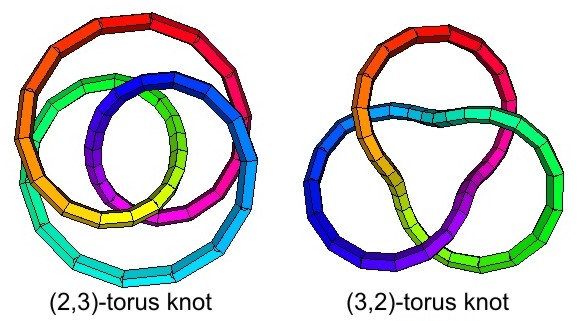
simple overhand knot, also called a "trefoil" knot. Above
is one
version, which mathematicians call "the (2,3)-torus knot toasted
with
cream cheese." But it is a bit tricky, so first try the
(3,2)-torus knot. They are both trefoils.

To start, you must visualize six
key
points. Three lie 120 degrees apart on the top of the bagel and
the
other three are directly below them on the bottom of the
bagel.
Then a smooth spiraling line connects each top point to the
other two
bottom points. For pedagogocal purposes, the construction
is
drawn with a marker on this bagel. For gastronomical
purposes,
you are advised to just visualize the points and lines and omit
the
drawing step.

Follow the line with a knife,
cutting
halfway in, and the cut should join with the opposite cut to
separate
through. You can gently work it open and see the bagel is now
one
continuous D-shaped band that goes three times through
the hole and two times around the hole. That is the meaning of
the
numbers in the notation "(3,2)-torus knot."
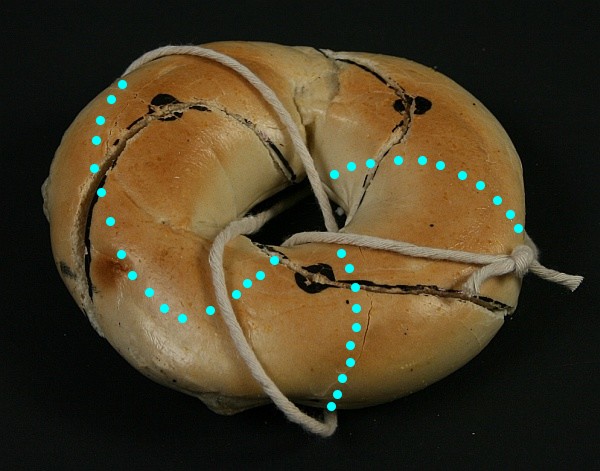
One way to convince yourself it
really
is a knot is to wrap a string along the surface, exactly
following the
path of the uncut bagel. Then tie the ends together to
form a
loop. (The string does not cross itself or the cut lines.)
Can
you visualize how the string forms a closed knot?

You have to break the bagel to
separate
it from the string. But then
you will clearly see the string has an overhand knot in it,
which means
the bagel did as well.

Now, on to the (2,3)-torus knot.
Again,
you need to visualize six key points. As the image above shows,
there
are just two dots on the top, 180 degrees apart.

The other four points are visible
from
the bottom. All six lie in one common plane. Each group of
three
lie 120 degrees apart on a circle that goes through the hole.
Again, a
smooth line connects each dot to the next, forming one
continuous
spiral.

This version turns out to be much
easier
to open up. (I think that is because it goes only two
times
through the hole, which is where things get tight.)

Here's the bottom view of the same
bagel. The path of the bagel goes three times around the
hole and
two times through it. Follow it around (or do the string thing)
to
convince yourself it really is an overhand knot.

Visualize the six points and the
curve,
so you can make the cut without drawing any lines. Then it is
ready to
pop into your Klein toaster and enjoy with a schmear of cream
cheese!
You can
explore torus knots and dream up many new bagel activities
with applets
such as
this.









