Card Constructions

George W. Hart




This page has ideas for two constructions that can be made with playing cards. In each case, the cards are just cut and interlocked without glue or tape.  The larger one, shown at right above, uses 60 cards.  The smaller one, at left, uses only 30 cards, but I think it may be harder to assemble. Together, the two can be made with two decks of cards.  The templates needed to cut the slits for these constructions are given below.


First Construction --- 60 Cards




This is an idea for a classroom activity to make a construction from 60 playing cards.  It is one foot in diameter.  Each card has four slits in it and they "simply" slide into their neighbors. No tape or glue is required, but the slits must be cut accurately for it to work well.

The underlying idea is an extension of the "Slide Togethers" which I have described here.  After seeing that page, Francesco De Comité had the idea to adapt the 30-square construction and make it with 30 playing cards. His result is shown here.  I think that is a great idea---I had thought of using old business cards and old post cards, but I'm not much of a card player so hadn't thought of using playing cards.  Cards are rather stiff, so they can hold their shape in something of a larger size as well. I thought it would be a good experiment to make a construction with twice as many cards, sixty.  (I am told that used cards can often be obtained free from casinos, so this is a way to recycle those cards.)




Above is the basic template.  It is proportioned for a 3.5 x 2.5 inch standard US poker card.  (If you use a bridge card, which is 3.5 x 2.25, just cut off a 1/8 inch strip from the left and right sides.) You can print this pdf file to have it in the exact size. I printed it to card stock, cut it out, cut out the slits, then traced the slits on to each card individually with a pencil, then cut the four slits on each card following the pencil line guides.




To assemble the cards, the tricky step is joining three together in a 3-fold lock, as shown above.  Notice the little equilateral triangle in the center. Each point is on top of the next, around in a cycle.  This is what holds everything from falling apart. I don't know how to tell you how to do this, but if you fool around and use the picture as a guide, you'll figure it out, I'm sure. A little bending is required during the assembly, but then each card becomes planar when done. Once you have mastered this lock joint, just join 20 of these 3-fold groups together as an icosahedron. You will form nice 5-fold stars with the slits at the other end of the card. The top image on this page is centered on one of the 5-fold stars.




It is worth noticing that the inside is quite pretty, like a kaleidoscope.

This is an experimental construction, which I have not tried out yet with anyone.  So I am not sure how hard it is.  But I envision it as a group project for 5 to 10 students to work on together in a class. If you try it, please let me know your ideas and suggestions. My plan is to develop the idea further with teachers at the Illuminations Summer Institute, then update this page.


Mathematical Ideas

There are a number of mathematical ideas that might be discussed in the context of this activity. Symmetry is one.  Students might be encouraged to locate and count the number of 3-fold axes, 5-fold axes, and 2-fold axes. The form can also be understood in terms of the polyhedron which underlies it.



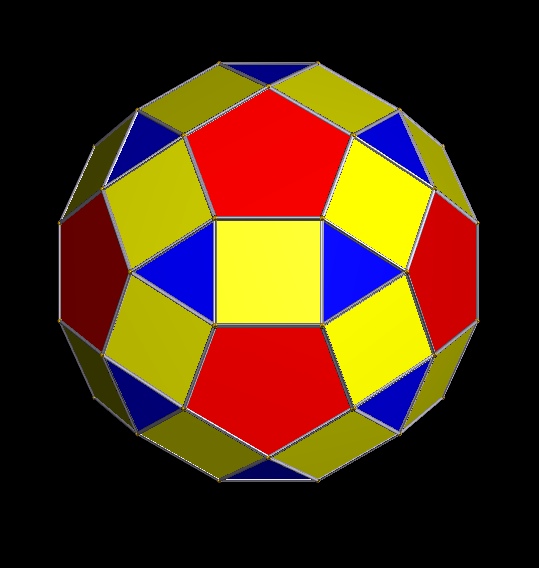
Above is a rhombicosidodecahedron, one of the Archimedean solids. It is "uniform on its vertices" , which means that every vertex is equivalent. At each vertex there is a pentagon, a square, a triangle, and another square. There are 60 vertices.




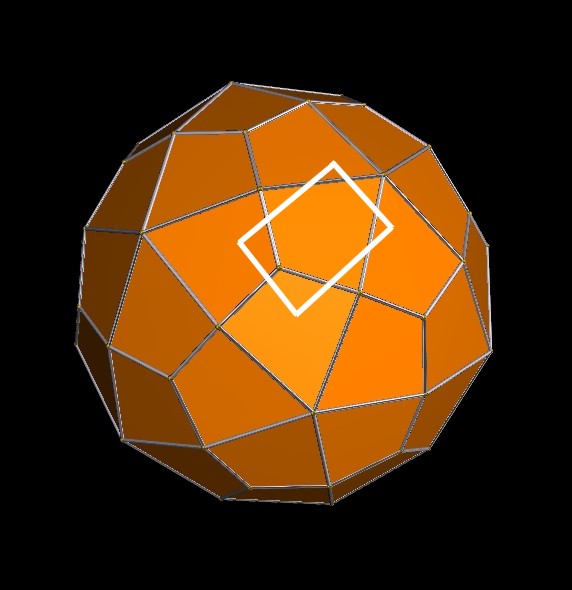
Above is the dual to the rhombicosidodecahedron. It has 60 faces. You can derive it (approximately) by putting a dot in the center of each rhombicosidodecahedron face. The four dots that surround any one rhombicosidodecahedron vertex become the vertices of one kite-shaped face of this dual.  So all the faces are equivalent. It is "uniform on its faces".  This polyhedron is called either the "trapezoidal hexecontahedron" or the "deltoidal hexecontahedron" depending on which book you check.  It is one of the Catalan polyhedra.

Our construction replaces each face with a card. The position of one card is sketched above, in white. It lies in the plane of one face, extending past the face at the corners of the card. Part of the card goes beyond the face at the 3-fold vertex, so when three cards overlap there, they lock. At the other end of the card, it does not quite reach the 5-fold vertex, so there are 5-fold openings in our construction. Also, the sides of the cards do not quite reach the 4-fold vertices, so there are 4-sided openings in our construction.



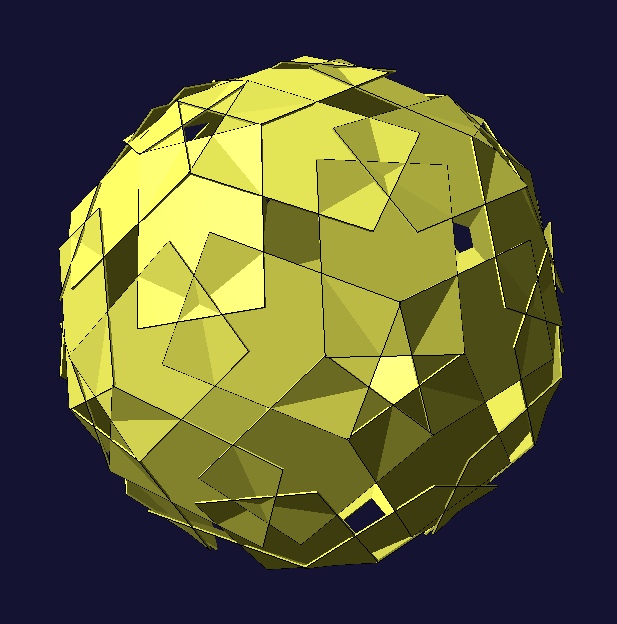
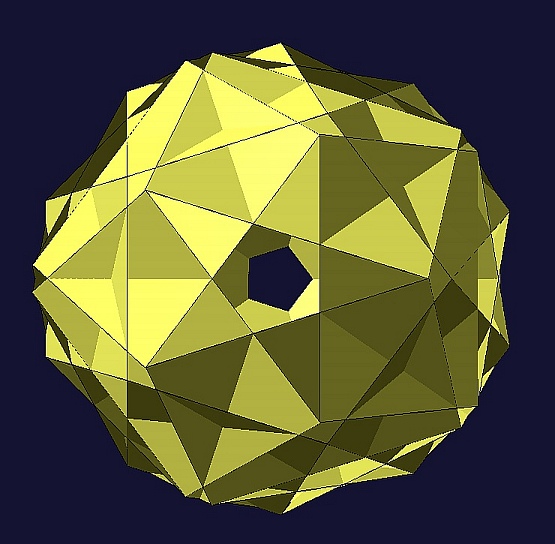
Above is a computer rendering of the construction.  I chose this polyhedron because it has 60 identical faces and the faces have bilateral symmetry.  But many other polyhedra can be adapted to this slide-together technique. That is left as an exercise for others.

Second Construction --- 30 Cards




This is an 8-inch diameter construction in which the 30 cards have more overlap, so it is trickier to make.  All the joints are 3-fold locks, so it holds together very tightly.  You can throw this around a room and it will not come apart, whereas the 60-card construction easily comes apart at its 5-fold stars.  But because the locks are deeper, it is probably not a good construction to try first.  The template to make your own is here. Both ends of the cards have equivalent cuts in this construction, so you don't have to worry about which end is which. If you already made the 60-card construction, this should be pretty straightforward once you figure out how to make one of these deeper 3-fold locks. 




I like the 5-fold stars which arise on the sides of the cards.  The geometry of this construction is the same as the slide-togethers with squares, except that I've used a rectangle instead of squares.  This requires that the relative size of the triangles and stars change.  I've make one particular choice for that change here, based on my aesthetic preferences. In Francesco De Comité's version, shown here, he made a different choice.  His has no 3-fold locks at all, as his cards are turned 90 degrees from my version.  So his has triangular openings instead of locks, and the cards are less obscured in his version.  This may make his easier to assemble, but also easier to fall apart. Take your pick or make both!




Here's a view looking directly at one of the twelve 5-fold stars.





This card-construction activity appeared in the Math Monday column on the Make Magzine blog from the Museum of Mathematics.