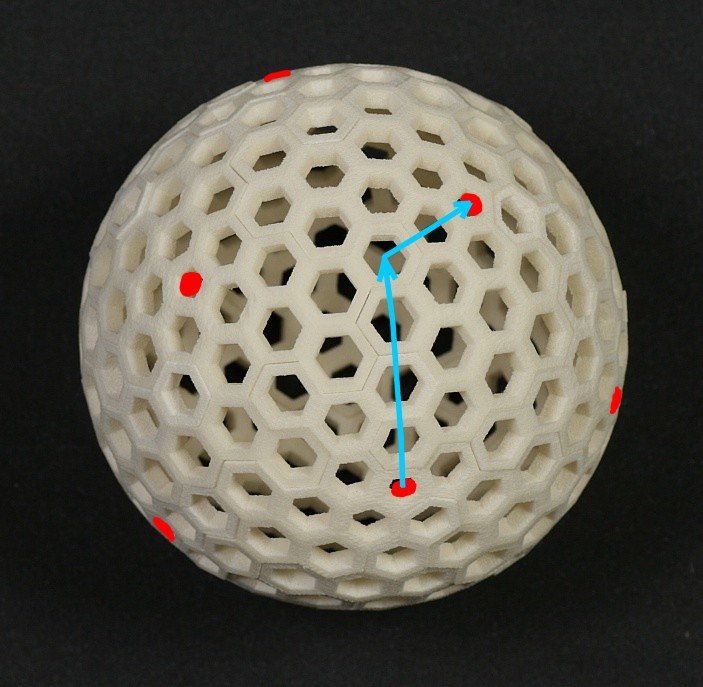
In the above image, the six pentagons in this hemisphere have been
colored red. (Six more pentagons are on the back
hemisphere.) The blue line indicates how the pentagons are
related. Start in any pentagon, facing any one of five directions, and
take four steps on hexagons. Then turn right 60 degrees and take two
more steps. You always land on another pentagon. That is the meaning of
the "4" and the "2" in calling this the (4, 2)-Goldberg polyhedron. The
mathematician Michael Goldberg worked out the theory of this family of
polyhedra in the 1930s. A nice example of how pure math ideas often are
later found to have useful applications is that since the 1980's these
forms are familiar to chemists as "Bucky balls".
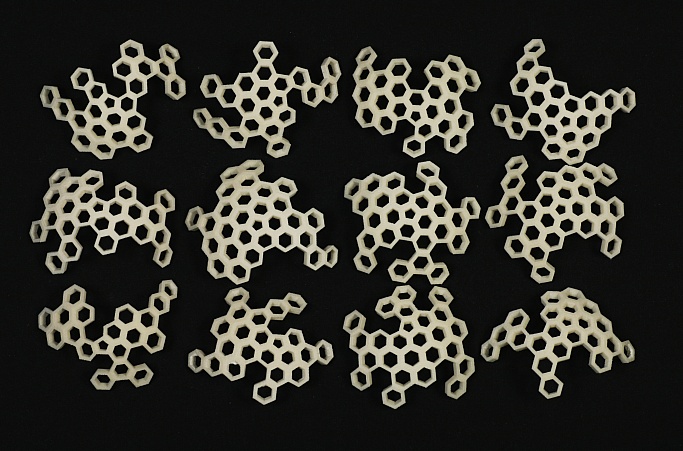
If you have access to a 3D printing
machine, you can make your own copy of this puzzle from the twelve stl
files which are available
here.
It
is
not
too
hard to assemble the pieces correctly if you remember to
check the (4, 2) property as you go along. That will eliminate
most of the incorrect matings which might initially look good. If
you get totally stuck, the six images above show how the twelve parts
go together.

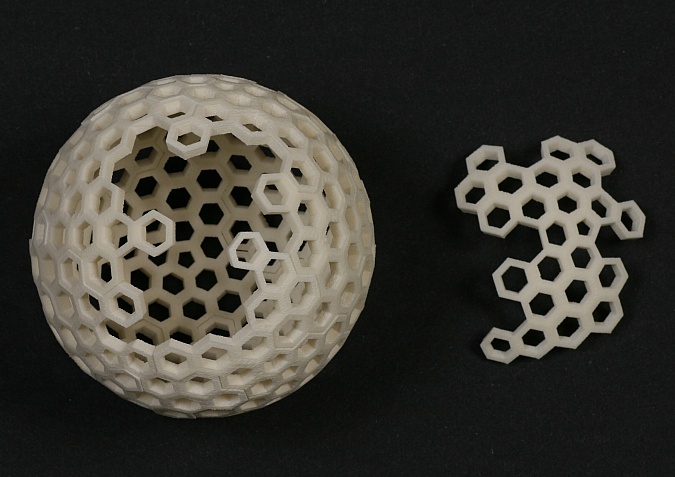
Above is a second instance of the same
puzzle. I dyed this one yellow and I think that helps make the
regions clearer, so when it is sitting on a shelf, people can see it is
a puzzle. It gives a nice sense of tectonic plates fitting
together, so I call this a Tectonic
Plates Puzzle. But geologists will no doubt point out that the
geometry of these regions is nothing like the geometry of real tectonic
plates.
In this image, the (4,2) puzzle is assembled and sitting inside a
slightly larger (5,3)-Goldberg puzzle which I made previously.
Pictures of that puzzle and the stl file for fabricating it are
available
here. As I get
time, I plan to continue this series of different concentric Goldberg
puzzles nesting inside each other.
For more about Goldberg polyhedra and other interesting uses for them,
see my
paper "Goldberg
Variations," to appear in
Shaping
Space.