Face-centered Cubic Voroni Constructions
George W. Hart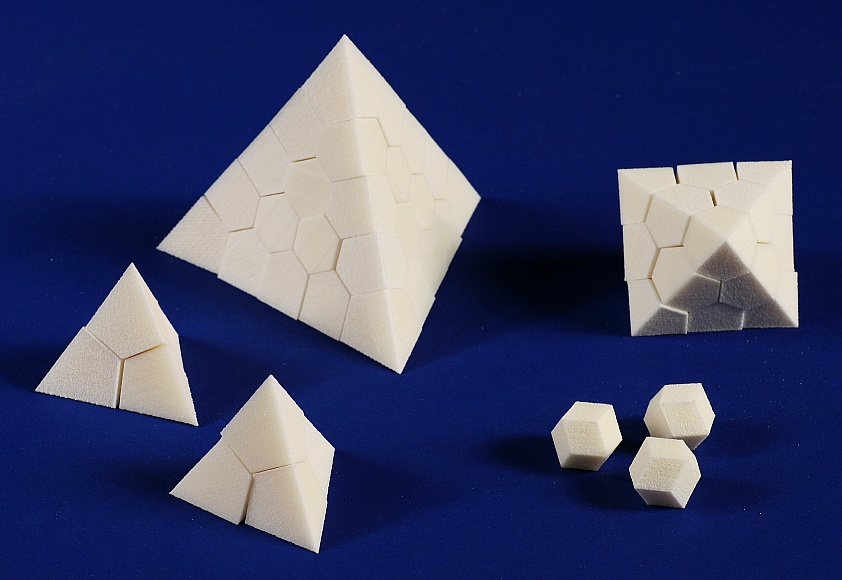
These are some solid constructions that
can be made with a small inventory of shapes for building blocks. The
idea is to take Voroni cells around points in a face-centered cubic
(FCC) lattice. Typical FCC cells are rhombic dodecahedra, as seen
at the right above. But we can slice through the FCC lattice in
natural places which define the space packing of alternating regular
tetrahedra and regular octahedra. When these polyhedra are
divided into Voroni cells by the FCC points that lie inside them, only
a small number of cell shapes result: interior cells, face cells, edge
cells, and vertex cells. The same units can be repeated to build
polyhedra of various sizes,
e.g., the four parts in the tetrahedron of size two at left above are
also
used as corners in the larger tetrahedron of size five. If we
properly position the polyhedral planes, then face cells for both
polyhedra are identical, and tetrahedra and octahedra can be combined.
It is fun to assemble these physical units. The octahedron is trickier
than the tetrahedron,
since gravity tugs on the parts in the overhanging faces.


The above image lets you see the
interior central rhombic dodecahedral cell in the size-five
tetrahedron. It also indicates how the tetrahedron and octahedron
can combine, e.g., to make a stella octangula.
The idea for this construction comes from a paper titled "Satterfield's Tomb" by David Klarner and Wade Satterfield (edited by Thane Plambeck) in the book A LifeTime of Puzzles: Honoring Martin Gardner, edited by Erik Demaine, Martin Demaine and Tom Rodgers. However, I am using a slightly different set of cutting planes than what is described there. (They propose only the regular tetrahedron and choose its planes as tangent to the spheres which inscribe the rhombic dodecahedra. I chose a parallel set of planes with "natural" integer intercepts, so one shape of face cells can be used in both the tetrahedron and the analogous octahedron.)
The cells above are made of nylon on a selective laser sintering machine. To minimize materials, I scaled these parts to be about 0.5 inches across, so they look like fancy sugar cubes. That works for me, as these images show, but you might prefer larger units for ease of handling.
If you have any kind of rapid prototyping machine, you can make your own cells by using these stl files:
The idea for this construction comes from a paper titled "Satterfield's Tomb" by David Klarner and Wade Satterfield (edited by Thane Plambeck) in the book A LifeTime of Puzzles: Honoring Martin Gardner, edited by Erik Demaine, Martin Demaine and Tom Rodgers. However, I am using a slightly different set of cutting planes than what is described there. (They propose only the regular tetrahedron and choose its planes as tangent to the spheres which inscribe the rhombic dodecahedra. I chose a parallel set of planes with "natural" integer intercepts, so one shape of face cells can be used in both the tetrahedron and the analogous octahedron.)
The cells above are made of nylon on a selective laser sintering machine. To minimize materials, I scaled these parts to be about 0.5 inches across, so they look like fancy sugar cubes. That works for me, as these images show, but you might prefer larger units for ease of handling.
If you have any kind of rapid prototyping machine, you can make your own cells by using these stl files:
- vertex of tetrahedron
- edge of tetrahedron
- face (of either)
- interior (of either)
- vertex of octahedron
- edge of octahedron