Sculpture of Morton C. Bradley, Jr.
George W. Hart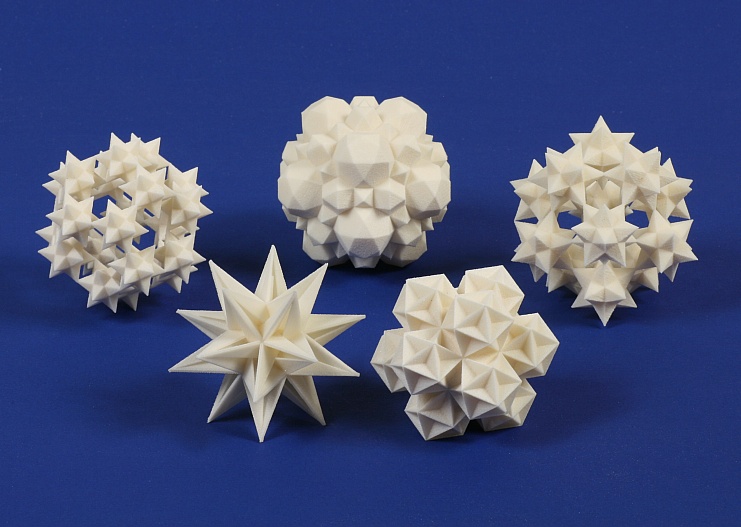
Morton C. Bradley (1912-2004)
was
well known as an art conservator but not sufficiently well
known for
his geometric sculpture. After his death, his large corpus of
sculpture
and models went to Indiana University. See the
IU
web
site for photos of his work and information about his
life
and the fabricators who worked with him to produce these
designs.
Most of Bradley's sculpture involved polyhedra-based constructions with color patterns based on the Munsell color sphere. I have re-created some of the forms with additive fabrication technology, addressing the geometry but not the color aspect of his work. This page briefly discusses five of his designs. Much more could be said, but my purpose here is to say just enough to get more people aware of and interested in his work. The stl files for the five models illustrated here can be downloaded, so anyone with access to a 3D printer can reproduce them. The stl files can also be viewed and rotated in an stl viewer to get a good sense of the 3D structure. Images of the five original Bradley sculptures below are used by permission of the Indiana University Art Museum.


The above model illustrates the form of Untitled 1971. It is composed of twelve corner-connected small stellated dodecahedra, centered on the twelve vertices of an imagined icosahedron. The original is 20" in diameter, made of 2-ply paper. The models shown on this page are each about 3 inches in diameter, made of nylon by selective laser sintering.
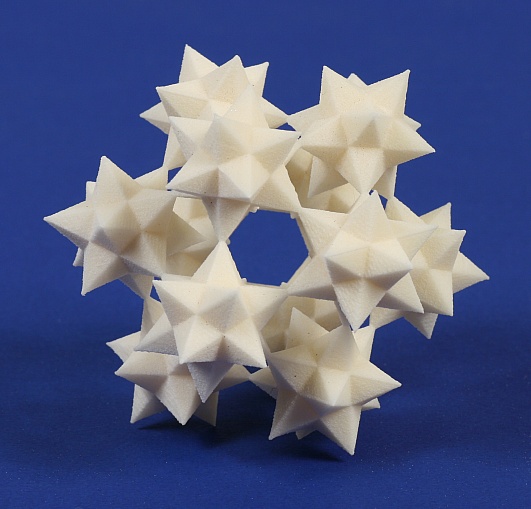
Like any good sculpture, this work has many different views, depending on the position of the viewer. In the above view, the camera is looking along a 3-fold axis. Compare it to the similar view in the IU slideshow. Ideally the units would each touch five neighbors at a mathematical point. Bradley's original paper sculpture could be held together and aligned by means of rigid wires that pass through the connection points. In these small versions, I approximate that by having the tips of the small stellated dodecahedra overlap their neighbors slightly. I tried to make the smallest possible overlap which allows the construction to hold together; so these models should be handled gently.
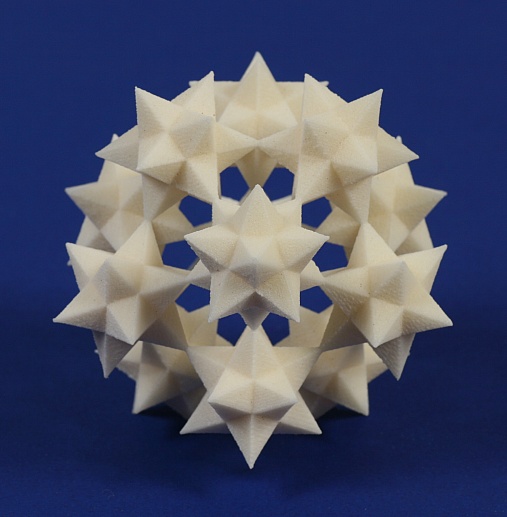
If one looks down a 5-fold axis, then Untitled 1971 appears as above. With access to a 3D printer, you can use this stl file to make your own copy.
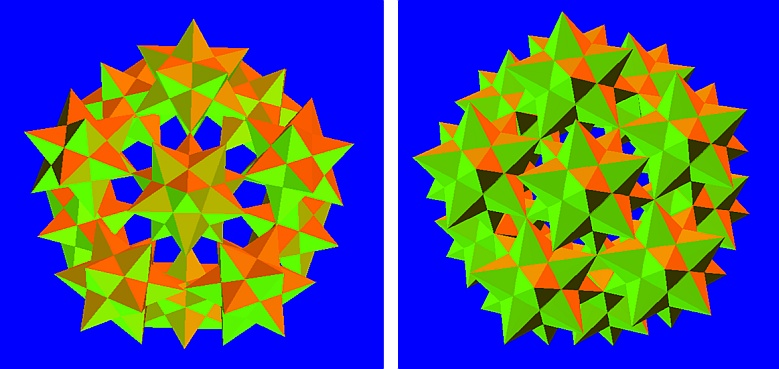
An important point to notice is that the twelve units of Untitled 1971 are not parallel. The rendering at right shows a similar form also consisting of twelve small stellated dodecahedra positioned at the vertices of an imagined icosahedron, but where the planes are parallel. In polyhedral assemblages of this sort, there are two possible orientations which preserve the overall rotational and mirror symmetry. These differ in that each unit in the more structured version at right is rotated 36 degrees relative to the corresponding unit in the more open version at left. Bradley chose the more directionally varied design on the left.
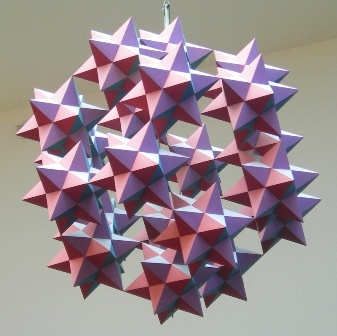
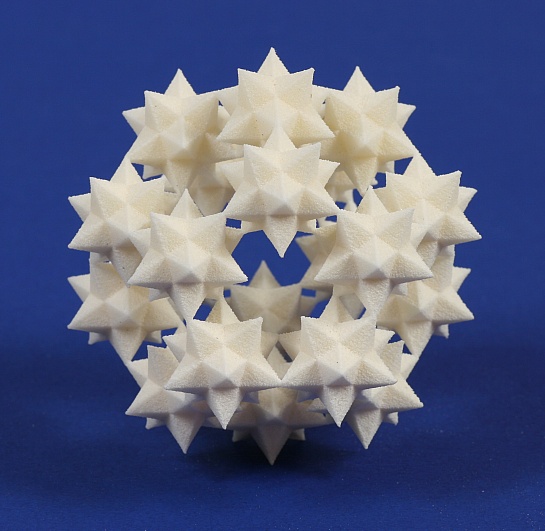
The form of Circus is also an assemblage of corner-connected small stellated dodecahedra. In this case there are twenty units instead of twelve. Each is centered on the vertices of an imagined dodecahedron. A photo of the original 24" paper sculpture can be found here. While Bradley's piece is designed to hang, a pleasing property of this structure is that it stands beautifully on three points (assuming you have a rigid, accurate model). So it has twenty built-in stands.
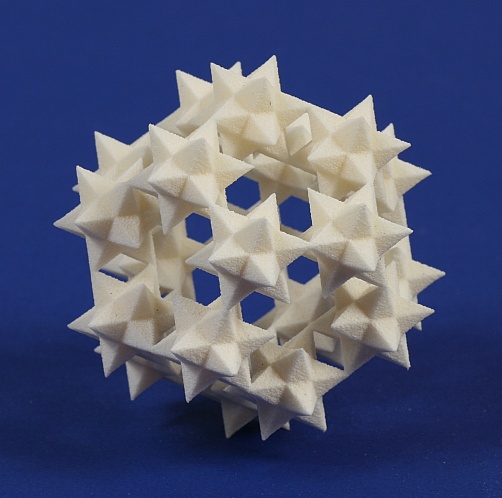
Again, different viewpoints have very different effects. In the above image, we look down a 3-fold axis.
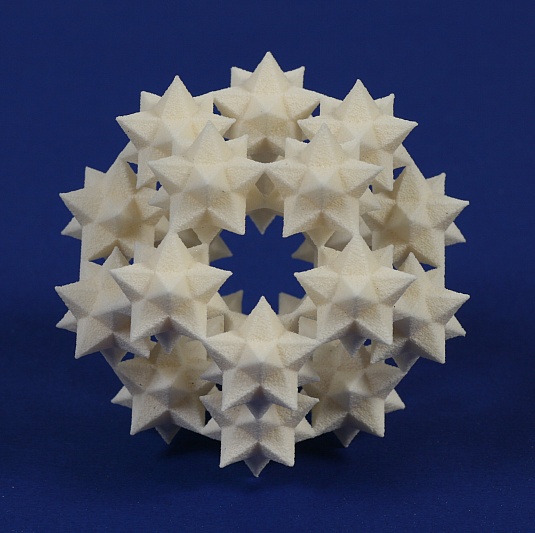
And above, Circus is viewed along a 5-fold axis. The stl file is here.
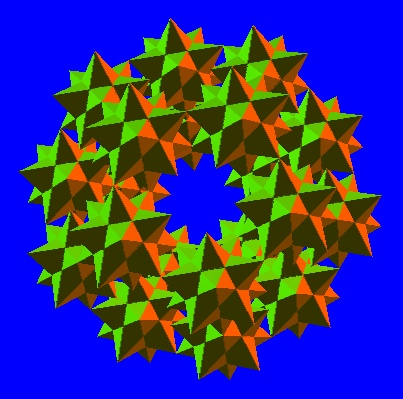

Again, there are two possible symmetry-preserving ways to choose the orientation of the components, as shown above. In the one at left above, all the units are parallel. At right, they have each been rotated 60 degrees, so are not parallel. Unlike Untitled 1971, for Circus, Bradley's version is the one in which the units are parallel.
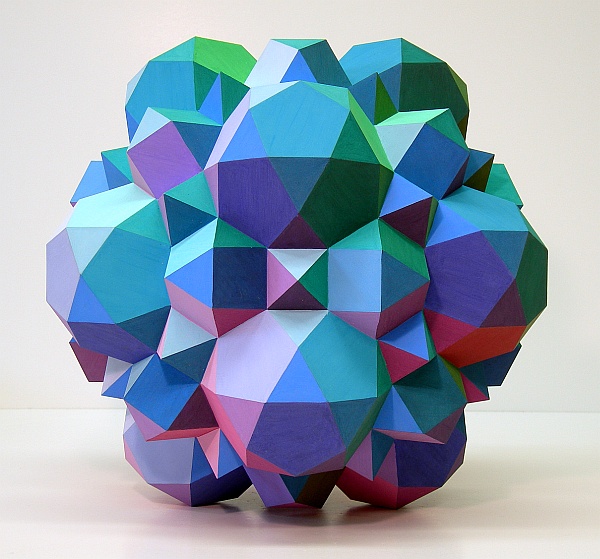

In a very different style, the form of Archimedes is based on a truncated icosidodecahedron which has been augmented with halves of icosidodecahedra, halves of cuboctahedra, and halves of octahedra. The original is painted plastic, 15" in diameter. It has a massive feeling when made in a solid color, but see the image in the IU slideshow to appreciate the very different visual impact the form acquires when painted in Bradley's color scheme. The stl file is here.
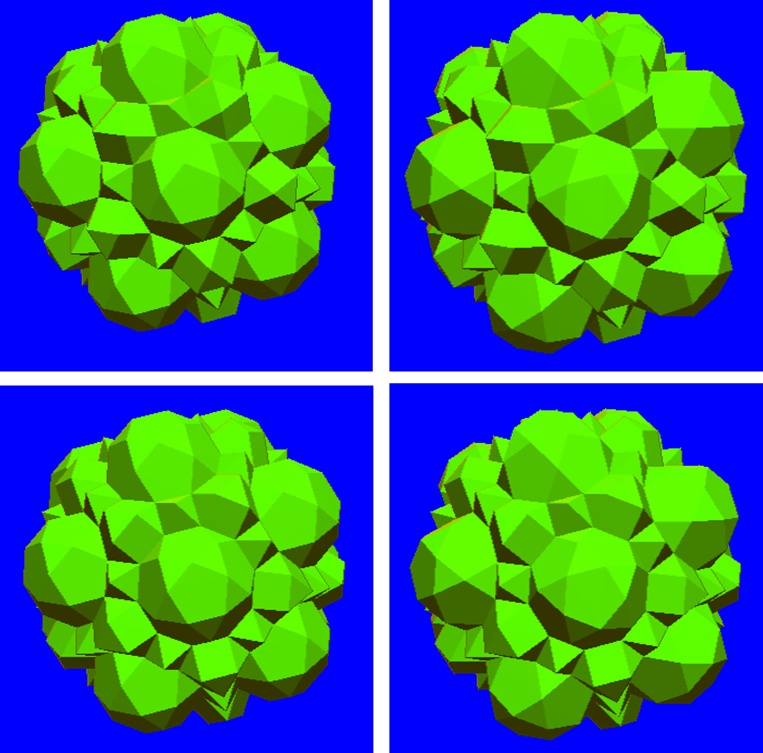
There are four different ways to attach the half polyhedra and still maintain the overall symmetry. Can you detect the subtle differences among these four and point out which Bradley chose?

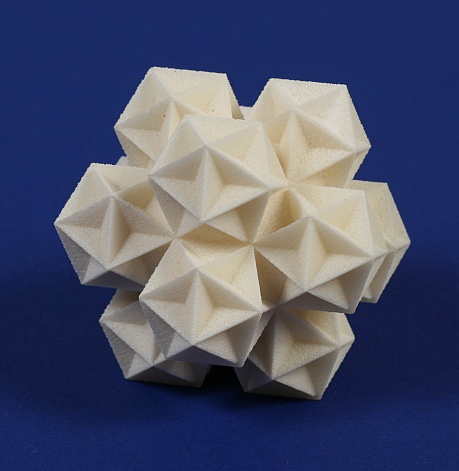
Untitled 1977 is an assemblage of twelve great dodecahedra joined along edges. Again, the centers are placed at the vertices of an imagined icosahedron. (I don't see a photo of this on the IU site to compare.) The stl file is here.

Above is the view along a 5-fold axis. Notice how the twelve modules each contribute one pentagon of a dodecahedron which seals off a space in the inteior.

It is interesting to visualize the shape of the trapped volume. It is like a dodecahedron with a star chiseled into each face.

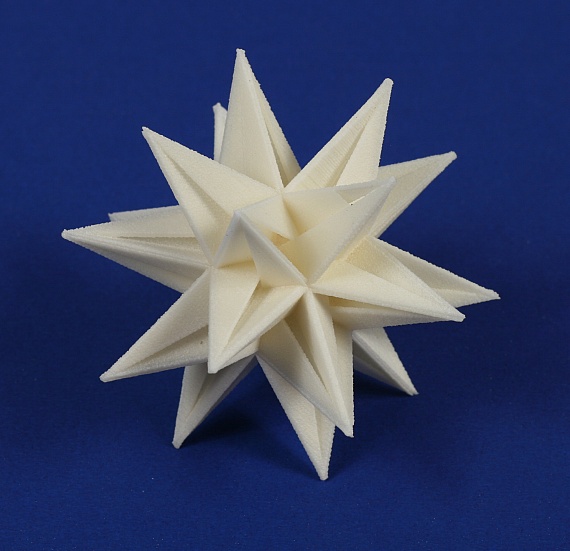
The form of Harlequin is based on a great stellated dodecahedron. It also does not currently appear to be on the IU web page, so I can not point you to a comparison image. One side of each star arm is cut away, as in Escher's Gravity. In its place is a triangular surface which connects that outer edge to the center of the form, as in Alan Holden's nolids.

Most of Bradley's sculpture involved polyhedra-based constructions with color patterns based on the Munsell color sphere. I have re-created some of the forms with additive fabrication technology, addressing the geometry but not the color aspect of his work. This page briefly discusses five of his designs. Much more could be said, but my purpose here is to say just enough to get more people aware of and interested in his work. The stl files for the five models illustrated here can be downloaded, so anyone with access to a 3D printer can reproduce them. The stl files can also be viewed and rotated in an stl viewer to get a good sense of the 3D structure. Images of the five original Bradley sculptures below are used by permission of the Indiana University Art Museum.
Untitled 1971


The above model illustrates the form of Untitled 1971. It is composed of twelve corner-connected small stellated dodecahedra, centered on the twelve vertices of an imagined icosahedron. The original is 20" in diameter, made of 2-ply paper. The models shown on this page are each about 3 inches in diameter, made of nylon by selective laser sintering.

Like any good sculpture, this work has many different views, depending on the position of the viewer. In the above view, the camera is looking along a 3-fold axis. Compare it to the similar view in the IU slideshow. Ideally the units would each touch five neighbors at a mathematical point. Bradley's original paper sculpture could be held together and aligned by means of rigid wires that pass through the connection points. In these small versions, I approximate that by having the tips of the small stellated dodecahedra overlap their neighbors slightly. I tried to make the smallest possible overlap which allows the construction to hold together; so these models should be handled gently.

If one looks down a 5-fold axis, then Untitled 1971 appears as above. With access to a 3D printer, you can use this stl file to make your own copy.

An important point to notice is that the twelve units of Untitled 1971 are not parallel. The rendering at right shows a similar form also consisting of twelve small stellated dodecahedra positioned at the vertices of an imagined icosahedron, but where the planes are parallel. In polyhedral assemblages of this sort, there are two possible orientations which preserve the overall rotational and mirror symmetry. These differ in that each unit in the more structured version at right is rotated 36 degrees relative to the corresponding unit in the more open version at left. Bradley chose the more directionally varied design on the left.
Circus


The form of Circus is also an assemblage of corner-connected small stellated dodecahedra. In this case there are twenty units instead of twelve. Each is centered on the vertices of an imagined dodecahedron. A photo of the original 24" paper sculpture can be found here. While Bradley's piece is designed to hang, a pleasing property of this structure is that it stands beautifully on three points (assuming you have a rigid, accurate model). So it has twenty built-in stands.

Again, different viewpoints have very different effects. In the above image, we look down a 3-fold axis.

And above, Circus is viewed along a 5-fold axis. The stl file is here.


Again, there are two possible symmetry-preserving ways to choose the orientation of the components, as shown above. In the one at left above, all the units are parallel. At right, they have each been rotated 60 degrees, so are not parallel. Unlike Untitled 1971, for Circus, Bradley's version is the one in which the units are parallel.
Archimedes


In a very different style, the form of Archimedes is based on a truncated icosidodecahedron which has been augmented with halves of icosidodecahedra, halves of cuboctahedra, and halves of octahedra. The original is painted plastic, 15" in diameter. It has a massive feeling when made in a solid color, but see the image in the IU slideshow to appreciate the very different visual impact the form acquires when painted in Bradley's color scheme. The stl file is here.

There are four different ways to attach the half polyhedra and still maintain the overall symmetry. Can you detect the subtle differences among these four and point out which Bradley chose?
Untitled 1977


Untitled 1977 is an assemblage of twelve great dodecahedra joined along edges. Again, the centers are placed at the vertices of an imagined icosahedron. (I don't see a photo of this on the IU site to compare.) The stl file is here.

Above is the view along a 5-fold axis. Notice how the twelve modules each contribute one pentagon of a dodecahedron which seals off a space in the inteior.

It is interesting to visualize the shape of the trapped volume. It is like a dodecahedron with a star chiseled into each face.
Harlequin


The form of Harlequin is based on a great stellated dodecahedron. It also does not currently appear to be on the IU web page, so I can not point you to a comparison image. One side of each star arm is cut away, as in Escher's Gravity. In its place is a triangular surface which connects that outer edge to the center of the form, as in Alan Holden's nolids.

There is a nice
pinwheel-like
chirality to the
form, as this view down a 5-fold axis shows. The stl
file
to make your own copy is here.

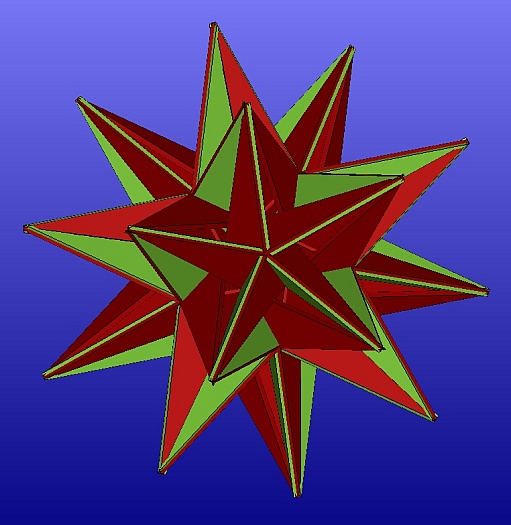
This two-coloring is not
the same
as Bradley's color scheme, but suggests a kind of jester's
hat, which
should make clear why the name Harlequin
is appropriate for the
structure.