George W. Hart

For a Museum of Mathematics fundraiser dinner, I created a series of mathematical table centerpieces.
These are each eight inches in diameter and built on the hi-def color ZPrinter model 650 from 3DSystems.
They vary in color and style, expressing different mathematical ideas in sculptural form.
Some, like the above, convey an organic sensibility, while others are more geometric.

This one has flower-like elements and stem-like arches on the interior within an overall organization based on the snub dodecahedron.
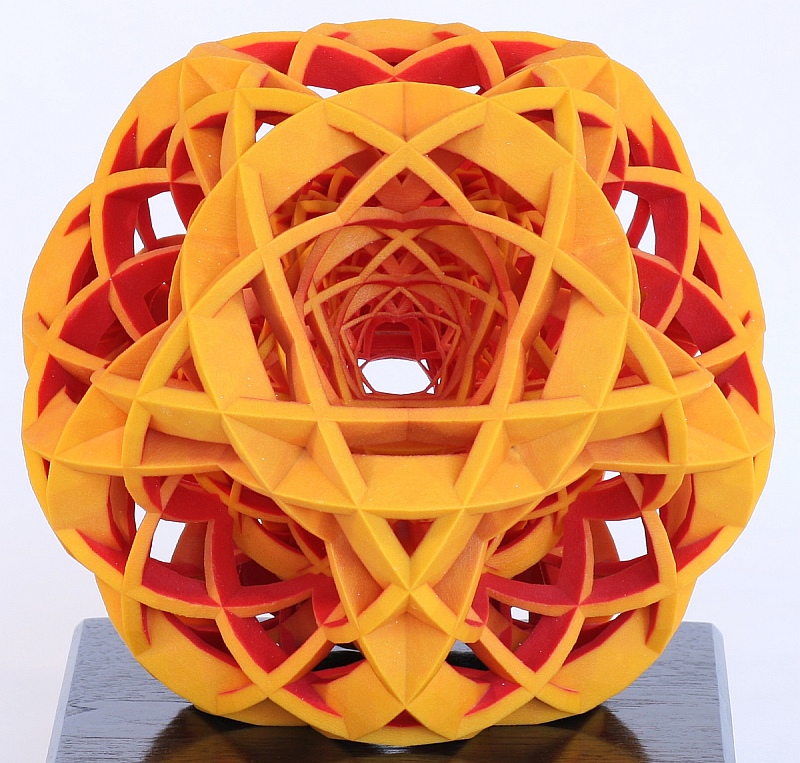
Here is a more geometric form, with nested copies of an arch-based structure.
Putting delicate elements on the interior protects them while giving a sense of depth.

This is another one with a biological flavor. It has a seedpod-like interior, from which pink star-pods grow.
The outer form suggests a small stellated dodecahedron, but with a chiral twist.

For those who prefer classic geometric designs, here is is one of my favorites, the compound of five regular tetrahedra.
These I made in a Leonardo style of open faces, encouraging a peek into the interior, with enough overlap to hold it all together.

Here again are five regular tetrahedra in five colors, but now they float in the interior space without directly touching.
They connect through the exterior rings and stars, which I lightened to pastel shades to help draw one's focus to the inside.

These sensuous forms convey a delicate warmth on the interior and a starfish-like icosahedral outside.
It is an updated version of my Mermaid's Delight sculpture.

One of my favorite four-dimensional forms is the 120-cell.
This baby-blue ball is based on the edges of a perspective transformation of the 120-cell.
Its 720 pentagons are rounded to circles and ellipses to give it a softer character (and more engineering strength).

Here is another centerpiece whose foundation is the 120-cell. This time the vertices are hollowed out.
The 10-fold appearance from certain special points of view is spectacular.
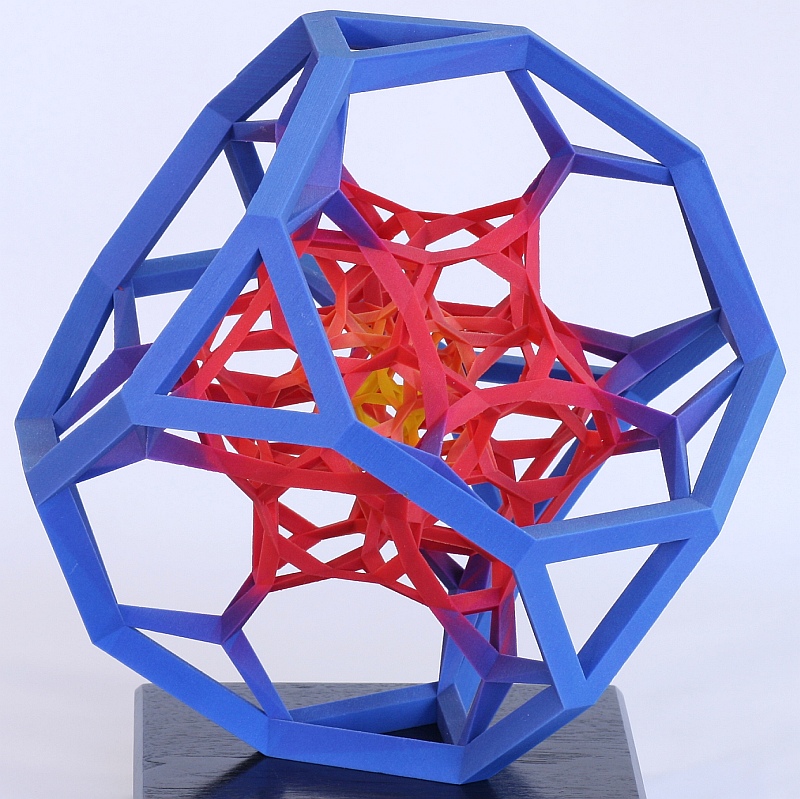
This centerpiece is based on the uniform compound of forty-eight truncated cubes, projected down from four dimensions to three.
I shaped it with a chiseled look, to emphasize its polyhedral basis and colored it with a yellow interior,
ranging through orange and red to a blue exterior, to give a sense of interior heat and energy.
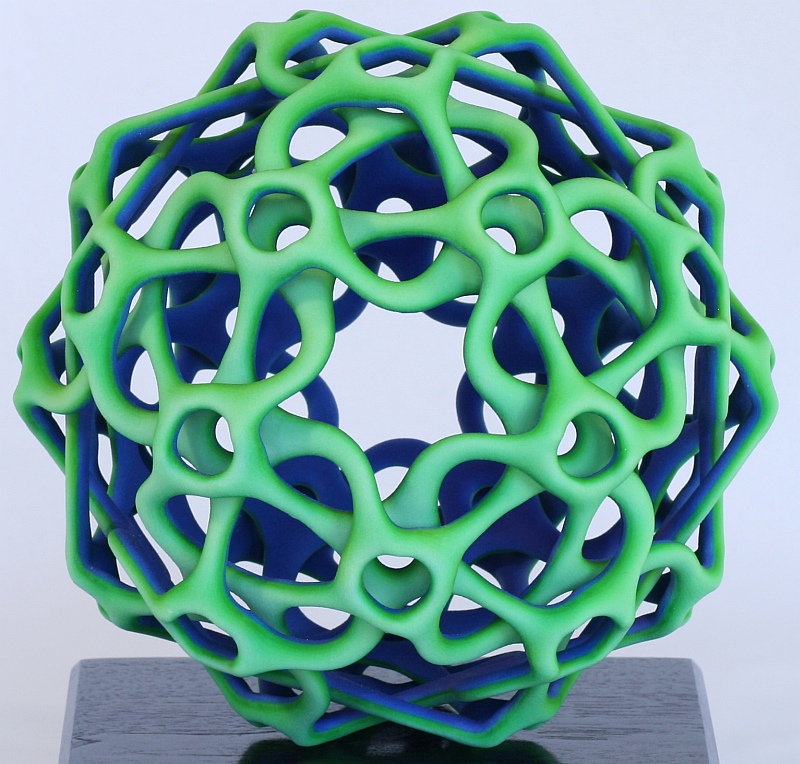
The inside and outside layers keep switching places in this knotted tangle.
It is an updated version of my Knot Structured sculpture.
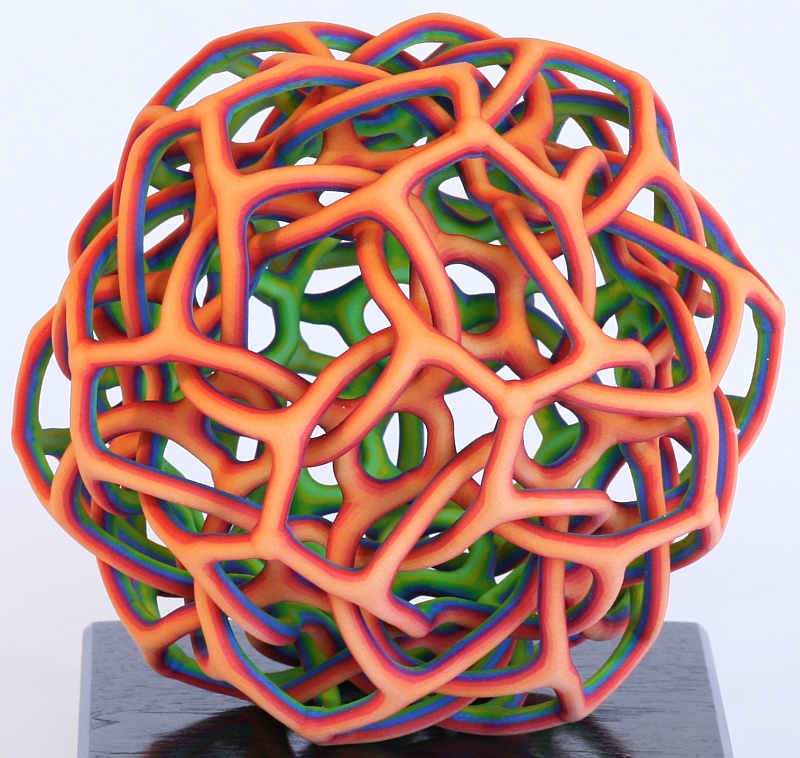
Taking the above idea further, here is an even more intricate tangle of edges, yet icosahedrally symmetric.
You can discover various knots if you follow the blue lines. (I was after a lizard-like coloring.)

Simple, yet worthy of study. (And not to be confused with a sepaktakraw ball.)
This is just the five regular tetrahedra again, but turned inside out!
Peek inside to see the 3-fold vertices.

Here, I was after a monumental architectural aesthetic (on an 8" scale). All the surfaces are portions of spheres.
(So all the cross sections as it was 3D printed layer-by-layer have circular boundaries.)

This one is a denser experiment with two interconnected layers and a surface that alternates between them.
Peering through the holes reveals its yellow interior.
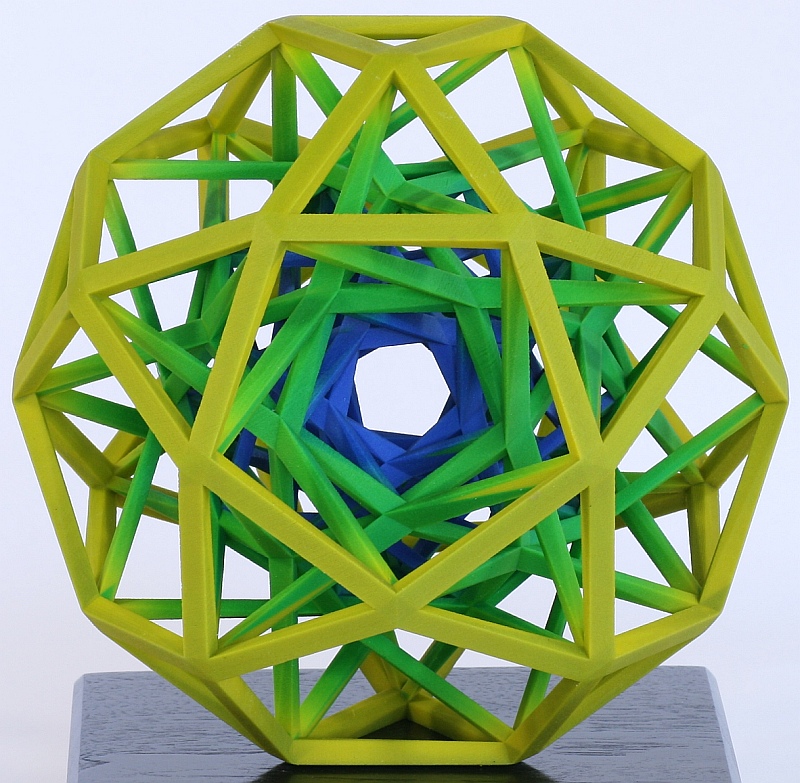
Back to a geometric vein, I like the spiral way in which these three nested icosidodecahedra connect.

For rainbow fans, here are six nested truncated cuboctahedra.
Again, the spiral way that each layer supports the next inner layer is worth a look.

This one is very cool for a number of reasons. What I did for the coloring is to make areas of positive curvature red,
negative curvature blue, and zero curvature yellow. Then I tweaked the shape until I liked the coloring pattern.

This undersea ballet pose consists of twelve starfish holding hands symmetrically.

Perhaps this conveys the feeling of a ball of fire, or solar storms, or some friendly virus.

Here are six sets of five parallel cylinders. Each set is orthogonal to a pair of dodecahedron faces.
This was a warm-up to the following one, which has twice as many rods.

Now pentagonal prisms alternating in two sizes each intersect eight prisms in other colors and the other size.
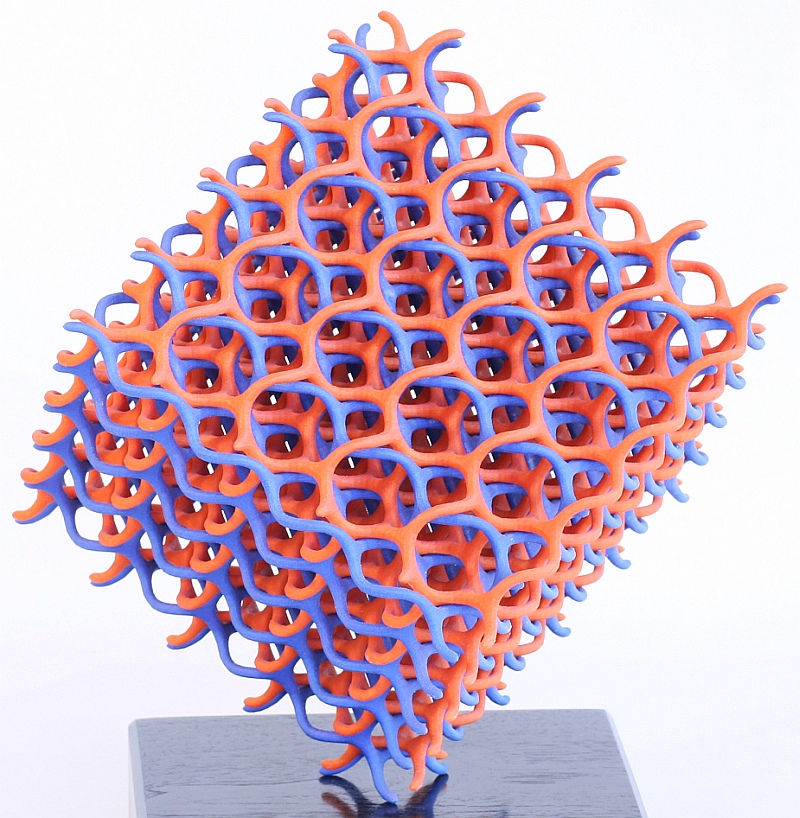
This consists of two interlocked lattices that are free to move slightly relative to each other.
It is a fascinating representation of the (10,3)-a lattice, explained here.
Thank you 3D Systems for supporting MoMath by making these centerpieces.