Dragonflies -- sculpture by George W. Hart

Here's a view down a three-fold symmetric axis.

Here's a view along a four-fold axis.

This is a 3.5 inch nylon model, made on a selective laser sintering machine.
It is assembled in 0.004-inch layers, which are clearly visible in the image.
(I made it the opposite chirality from the wooden version.)

Mathematical Derivation

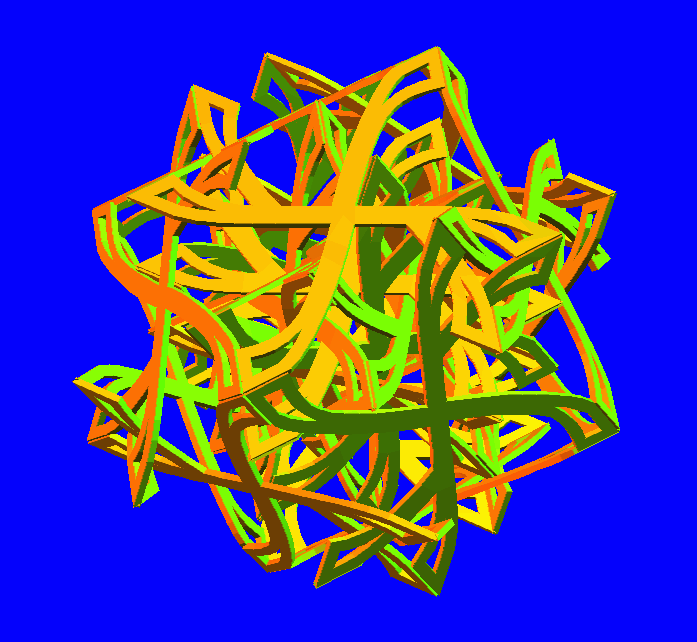
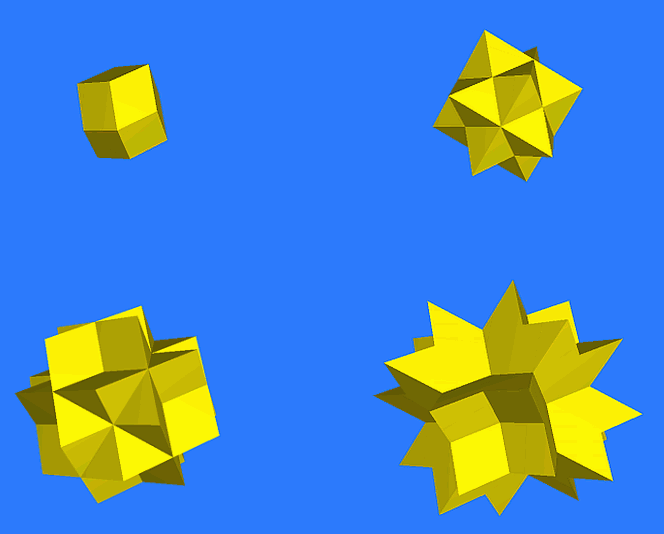
The design for Dragonflies derives from the third stellation of the rhombic dodecahedron (RD). The image above shows the RD in the top left and its three (successively larger and more complex) stellations. The RD consists of twelve rhombic faces. The RD stellations are derived by extending the RD's face planes symetrically until they meet and enclose a volume outside the inner polyhedron. Much of each face plane is hidden inside the outer shell; we only see certain facets of the faces in the stellations above. These four forms have many interesting geometric properties, e.g., the first two are space-fillers and they all have been used as the basis of mechanical puzzle designs.

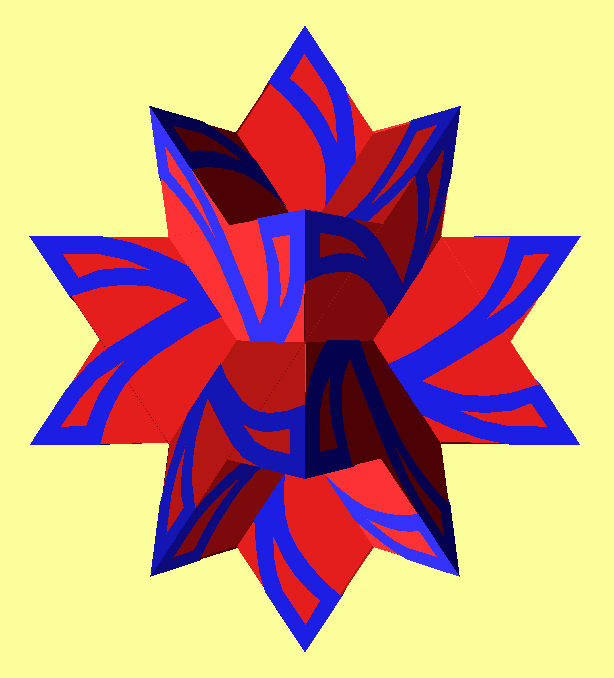
The wooden components of Dragonflies are designed as a subset of the complete face of the third stellation of the RD. The image above shows how that subset lies within the complete face plane. The subset was designed so that it does not intersect with the other eleven copies of itself. Interweaving and assembling the rigid physical parts is an interesting challenge.

Assemblages

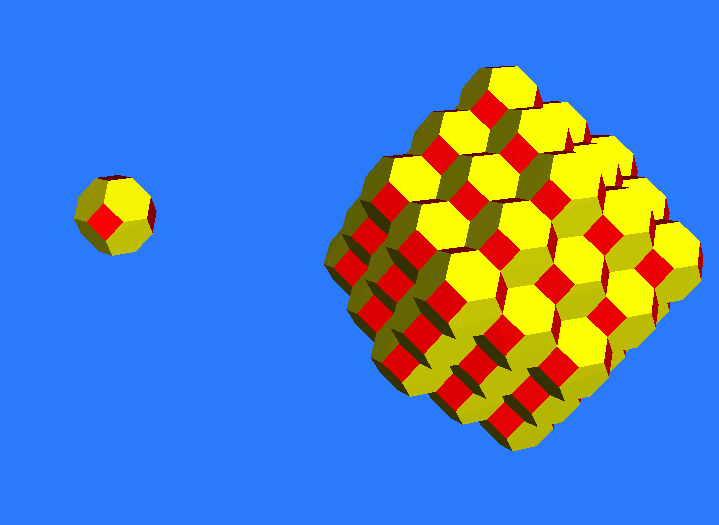
The convex hull of the third stellation of the RD is the truncated octahedron, which is the Archimedean solid with two hexagons and one square at each vertex, shown at left above. This is a space-filling polyhedron, which means it packs together with copies of itself to fill space, as indicated at right above. So copies of the Dragonflies sculpture can be assembled together to form three-dimensional networks.

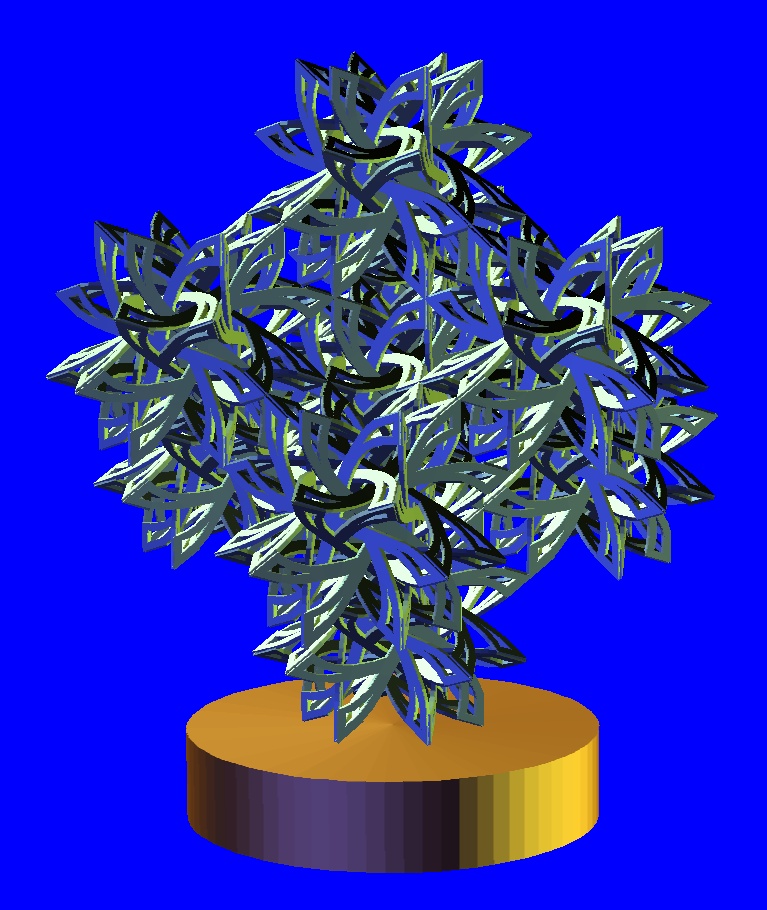
I have a number of designs in mind for possible large-scale sculptures based on these concepts.
The above consists of nine units, outlining a cube standing on a corner, totalling of 108 dragonflies.

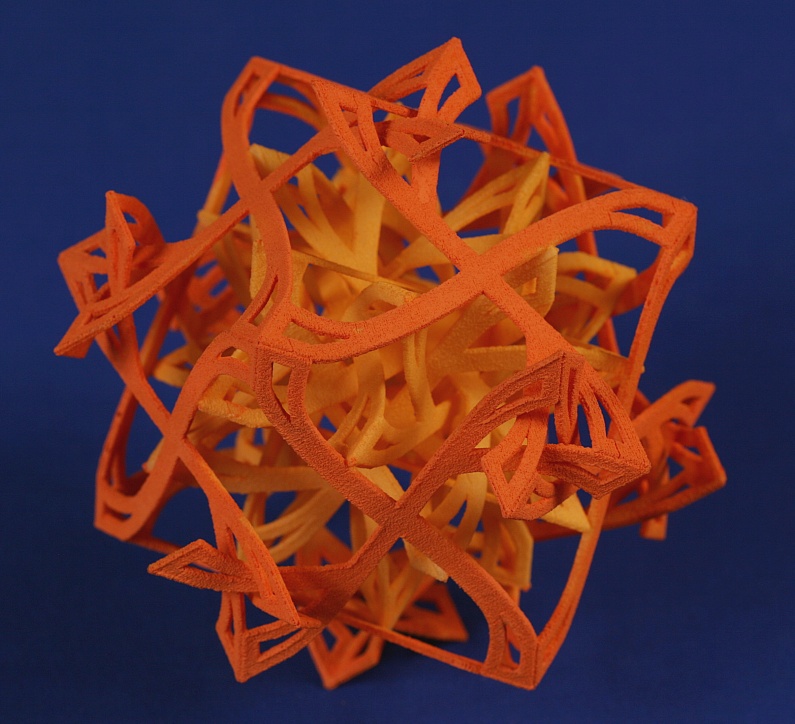
Here is a 3.5 inch nylon model of this design.

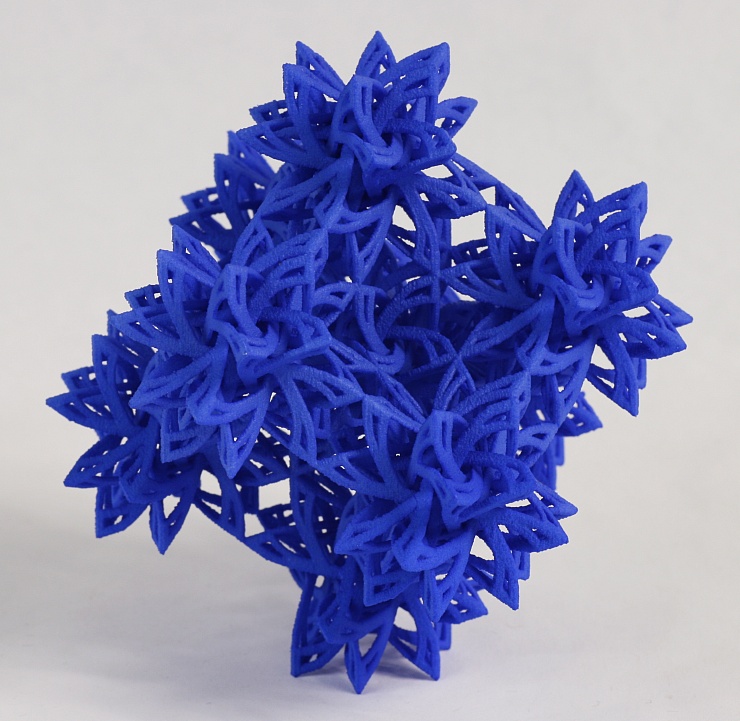
Here it is again, in blue.

And here is a similar assemblage of just six units, totalling 72 dragonflies.

Second Configuration

A very interesting fact is that these same twelve components can be assembled in a second configuration.
The components here have been translated radially outward, but not rotated.
They each connect to others in the same places, but to different neighbors.

This image shows both configurations together, arranged concentrically. Observe that for each part
of the original inner configuration, there is another part exactly parallel to it in the outer configuration.
This is very interesting geometrically and conceptually, but in one color it can be hard to understand.

This is a rapid prototyping model of the combined design.

It is a bit obtuse from certain points of view, but from others it quite interesting visually.

I especially like this 3-fold view.

Using two colors brings out its structure nicely so you can see the two concentric layers.
I dyed it all yellow then painted the outer parts orange. I call it Orange and Yellow.