The
Zometool (officially Zome System) is a plastic construction set
which lets you quickly and easily build edge models of many complex polyhedra.
(A link to the manufacturer is at the bottom of this page.) Building your
own is the best way to really understand anything and Zometool models can
go far beyond what you can build as paper models.
Note that the actual 3D models are much clearer than the 2D images on this
page might suggest, since here you are seeing the front and rear superimposed.
The
Zometool (officially Zome System) is a plastic construction set
which lets you quickly and easily build edge models of many complex polyhedra.
(A link to the manufacturer is at the bottom of this page.) Building your
own is the best way to really understand anything and Zometool models can
go far beyond what you can build as paper models.
Note that the actual 3D models are much clearer than the 2D images on this
page might suggest, since here you are seeing the front and rear superimposed.
Any structured construction set has certain advantages and disadvantages.
For example, there are only certain angles and lengths built in to the
Zometool, so certain objects can not be constructed. On the other hand,
the edges and angles available have been very cleverly designed as the
symmetry
axes of the icosahedron/dodecahedron. The range of things which can
be built with edges in these directions is quite amazing and far beyond
any other construction set I know. Furthermore, the structured design of
the parts guides you into making things that you didn't know you could
make.
The basic struts (edges) come in three colors, and each color comes
in three lengths. Red edges are parallel to 5-fold axes, yellow edges are
parallel to 3-fold axes, and blue edges are parallel to 2-fold axes. The
struts and the connector holes have 5, 3, and 2 sides to make this work
out automatically. The lengths are scaled by the golden ratio so that if
you make a pentagon of one size strut, the next larger size can fit in
as its diagonal. In getting to know the set, this all becomes very natural.
One tip is worth emphasizing however: Get in the habit of pushing the struts
completely into the nodes or later your larger models will fall apart.
 |
The
icosahedron and dodecahedron
are natural projects to begin with. In a few minutes anyone can build one,
regardless of prior geometric experience. You can either build them directly,
or use a two-step process for your first time. The two-step process to
build an icosahedron starts with you putting a red strut into all twelve
5-sided holes of one ball. Balls placed at the ends of these red struts
are the twelve vertices of an icosahedron. You then connect these to each
other with blue struts. Having done this once, you will find it easy to
build just the icosahedron edges without the central red scaffolding the
next time. |
(Incidentally, if you look at an icosahedron with internal
red struts, you see that a solid icosahedron can be dissected into twenty
solid tetrahedra which meet at its center. Note however that the tetrahedra
are not regular tetrahedra as some people erroneously assume. The
fact that some edges are red and some are blue should make clear that they
are not all the same length.)
The two-step process for the dodecahedron
is analogous, but start with twenty yellow struts in the center ball to
locate the twenty vertices of the dodecahedron. Again, after doing this
once, it is easy to eliminate the scaffolding and directly build just the
dodecahedron the next time you do it.
| With soon-to-be-unveiled green struts, the regular tetrahedron
and octahedron are also constructible.
You can also make many irregular tetrahedra and octahedra. The tetrahedron
shown is one twentieth of the icosahedron built above. The octahedron in
the middle has eight identical faces, but they are isosceles, not equilateral.
The octahedron at right has two equilateral faces and six isosceles. |
 |
 |
The remaining Platonic solid, the cube,
is borderline buildable with Zometool. Just make a square from blue struts,
and your cube will arise naturally. However, careful observation reveals
that this is not the familiar cube which it mimics. A real cube has three
axes of 4-fold symmetry, whereas the Zometool connector nodes do not allow
true 4-fold symmetry. This is really a "pseudo-cube" with tetrahedral symmetry
and only three mirror planes (not nine
as in a real cube). The orientation of the blue edges has two up and two
flat in each square, so each square really has only 2-fold symmetry. This
may seem like a minor subtlety, but the whole point of the Zometool is
really to understand symmetry axes, so it is worth pausing to observe that
this cube (and the octahedron) does not have true 4-fold symmetry. |
| While you are making the Platonic solids, it is a good exercise to
build a model of a cube inscribed
in a dodecahedron. (It is clearer in person that this photo suggests.)
Either start with a dodecahedron and add one diagonal to each face, or
start with a cube and add the dodecahedron's edges as a kind of "roof"
on each side. The cube edge is a diagonal of the pentagon, so it is the
golden ratio times as long, i.e., the next longer size blue strut. |
 |
For a project to illustrate duality, construct
a compound
of an icosahedron and a dodecahedron which cross each other at their midpoints.
You can do it with the dodecahedron edge being two short struts and the
icosahedron edge being two medium struts.
 |
Among the Archimedean solids, five
with icosahedral symmetry can easily be constructed using only blue struts.
Six more can be made with the green struts. (The snub
icosidodecahedron and snub cube
are not Zometool-constructible as their edges are not parallel to symmetry
axes.) At right is the truncated
icosahedron, sometimes known as a soccer ball, or buckeyball. |
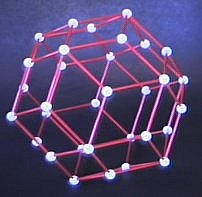
| Here is a rhombicosidodecahedron.
This is the same general shape as the Zometool connector ball. If you use
two sizes of struts, stretching the squares into rectangles and enlarging
the triangles, you can make a large model of the Zometool ball |
 |
| The second Archimedean dual which you can make is the rhombic
dodecahedron. Analogous to the cube above, this is really a "pseudo-rhombic
dodecahedron" without true 4-fold symmetry if you look closely. Try adding
blue struts as the short diagonal of each face, to see how a
cube is inscribed in the rhombic dodecahedron. Or add green struts
as the long diagonals to see the octahedron
inscribed in it. Being a space-filling polyhedron, you can extend your
rhombic dodecahedron model with more copies of itself which fit face-to-face
and fill all space without gaps. |
 |
 |
The above two Archimedean duals happen to be zonohedra.
Many other zonohedra can be constructed with the Zometool, as long as their
edges are parallel to the some of the 31 axes of symmetry of the icosahedron.
For example, this rhombic enneacontahedron
is based on the 3-fold axes of the icosahedron. |
Many other zonohedra based on the icosahedral symmetry axes can be constructed
with the Zometool. For example, try making a (red and yellow) 16
zoner, a (red and blue) 21
zoner, or a (yellow and blue) 25
zoner The ultimate is the
compete 31-zone zonohedron based on all the icosahedral axes.
| After mastering zonohedra, you might like to try some of my zonish
polyhedra. Victoria is resting at right, eating an apple, inside a
structure
she just made. |
 |
 |
And Colin can be seen at left, inside a zonish structure
he built. |
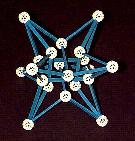
| Next we come to stellations. Here
is a very nice one of the
tetrahedral stellations of the dodecahedron. This one is especially
interesting because it can fill space if you alternate it with regular
dodecahedra. If you think of the model of a cube in a dodecahedron above
as a cube with six roofs attached, then this is the same cube with the
same six roofs subtracted from its interior. |
 |
 |
The real fun of the Zometool is that it lets you doodle in three-space.
Here is one example, but if you have made many of the above, I am sure
you have already many of your own doodles. This structure is based on the
dodecahedron, but each edge is replaced by two yellow struts which make
an outward pointing "V". |
Going beyond three dimensions to four, a wonderful model is this projection
of the 4-dimensional 120-cell into 3 dimensions. Pictures of a wire model
of this structure, made by Paul Donchian, can be found in Coxeter's Regular
Polytopes and in Banchoff's Beyond the Third Dimension. You
have to see it or make it yourself to really appreciate it. Start with
a medium-sized dodecahedron as its core; the result is about 1 meter in
diameter:

Details on all the above, lots more new examples, and lots of real geometry
(lengths, angles, volumes, theorems, etc.) are now available in our comprehensive
book:
The book and the Zome kits can both be ordered from either the Zome
Corporation or Key
Curriculum Press. You can also try internet toy sellers like
Amazon.
Many of the models above were made by 8-, 10-, and 12-year-old Victoria,
Colin, and Christopher.
 The
Zometool (officially Zome System) is a plastic construction set
which lets you quickly and easily build edge models of many complex polyhedra.
(A link to the manufacturer is at the bottom of this page.) Building your
own is the best way to really understand anything and Zometool models can
go far beyond what you can build as paper models.
Note that the actual 3D models are much clearer than the 2D images on this
page might suggest, since here you are seeing the front and rear superimposed.
The
Zometool (officially Zome System) is a plastic construction set
which lets you quickly and easily build edge models of many complex polyhedra.
(A link to the manufacturer is at the bottom of this page.) Building your
own is the best way to really understand anything and Zometool models can
go far beyond what you can build as paper models.
Note that the actual 3D models are much clearer than the 2D images on this
page might suggest, since here you are seeing the front and rear superimposed.