

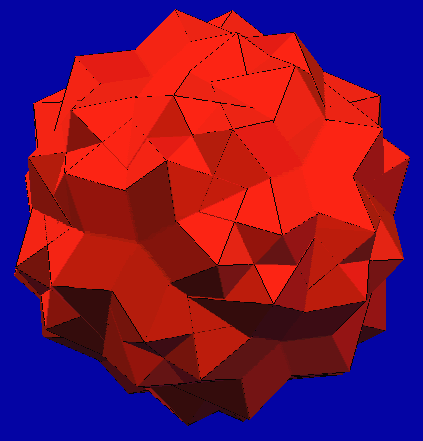
The uniform compound of five icosahedra
The anti-zomeball has a wonderful non-obvious property: its 60 vertices are at the positions of the vertices of five regular icosahedra. The image above shows the uniform compound of five icosahedra. Study it to see that there are 12 concave five-pointed-stars. Their 60 vertices are arranged exactly as in the 12 pentagons of the anti-Zomeball. One way to think of the compound is to relate it to the compound of five cubes (or the compound of five octahedra,) and inscribe one icosahedron (properly oriented) in each cube or each octahedron).
You can put a small yellow strut as a marker on 12 balls of the anti-Zomeball, to indicate the vertices of one icosahedron. But sadly, most of the edge directions of these five icosahedra are not Zome constructible, so we can locate their vertices (as shown at the top of the page), but we can not build the edges with Zome struts. However, if one is patient, one can form the edges of the five icosahedra by running five colors of thread between the balls. Just use a needle, as when sewing. (I haven't yet made this with all five icosahedra. If you do, send me a photo to insert here.)
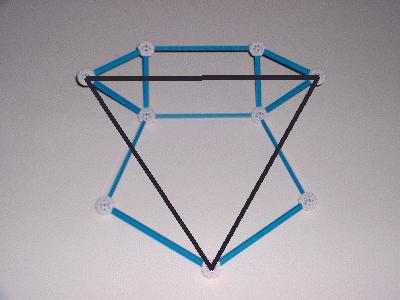
This image shows how to thread one face of the icosahedron. The
interesting fact which makes this an exact construction is that the two
types of vertex-to-vertex distances indicated with black lines in the image
above are exactly equal. (In the model shown, with b1 x b2
rectangles, the icosahedron edge length is 2b2.) Exercise:
Using the coordinate methods of Chapter 18, show that the edge which crosses
a triangle, a rectangle, and a triangle has equal length to the edge which
crosses the rectangle and the pentagon.
 |
 |
|
small stellated dodecahedra |
great dodecahedra |
The vertices of the icosahedron are also the vertices of the small stellated
dodecahedron and the great dodecahedron. (See Chapter 20 of Zome
Geometry.) So by replacing the five icosahedra with either of
these polyhedra, we obtain two additional uniform compounds. The
two figures above show how their faces intersect. Notice again how
their 60 vertices are identically arranged. Again, their edges are
not all in Zome directions, so these compounds are not Zome constructible,
but either can be made with thread if you are patient and careful.
The gread dodecahedron has the same edges and vertces as the icosahedron,
so if you make a thread model of the five icosahedra, it is also a model
of the five great dodecahedra. The figure at the top of this page
shows one of the five small stellated dodecahedra made from white thread;
look for its 12 pentagrams. Adding the other four small stellated
dodecahedra, in four other colors of thread, should create a nice model
of the compound at left. (Again, I haven't made a complete model;
if you do send me a photo to put here.)