|
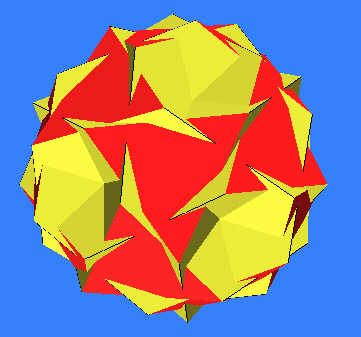
Image of compound of six pentagonal prisms, showing how
the faces intersect. (The green faces are pentagons; the blue faces are
squares.)
|
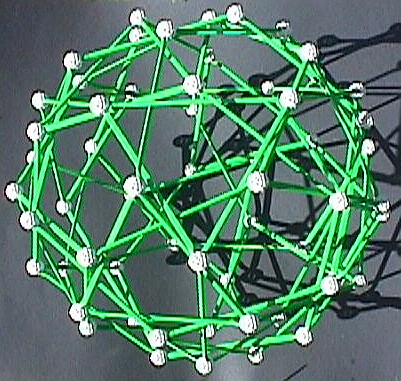
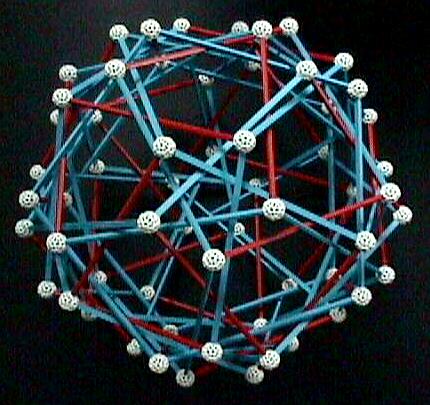
Zome model of compound of six pentagonal prisms, requires
60 b3 struts and 30 r3 struts. (The prisms' sides are
rectangles, not quite squares.)
|