Additions to

Zome Geometry

Hands-on Learning with Zome Models

George W. Hart   and  Henri Picciotto


Zome Snowman

Here is a "snowman" made from three stacked polyhedra.  Each ball is based on the truncated icosahedron---the familiar shape of the soccer ball.  They are connected to each other pentagon-to-pentagon, and the whole structure has a vertical five-fold axis of symmetry.  Start at the bottom and work up:

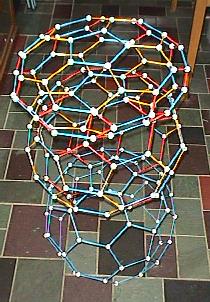
The bottom ball is the standard truncated icosahedron, made with b2s.  It has a pentagon at its top, and is cut off at a point which gives a stable base.  Five b3s are used in the base polygon.  (They cut the diagonals of what would otherwise be five pentagons.)

The middle ball is topologically a complete truncated icosahedron, but is compressed to be somewhat oblate. It has a b2 pentagon at its bottom and top, then b2-r2-r2-b2-r2-r2 hexagons surrounding those, then b2-y2-r2-r2-y2 pentagons in the next layers, and b2-y2-y2-b2-y2-y2 hexagons zigzaging to form the equator.

The top ball is another truncated icosahedron, squished even further.  It also has a b2 pentagon at its bottom and top, but then b2-y2-y2-b2-y2-y2 hexagons surrounding those, then b2-r1-y2-y2-r1 pentagons in the next layers, and then b2-r1-r1-b2-r1-r1 hexagons zigzaging to form the equator.

At right is another view, which shows the top pentagon and its neighboring hexagons more clearly.

To understand the squished balls, it helps to have made the 120-cell model (Unit 21.4) and realize that these two squished truncated icosahedra are analogous to the 120-cell's projected dodecahedra of type 2 and type 4.

You can also make a truncated icosahedron squished along a 3-fold axis, so it has a regular b2 hexagon at top and bottom; it corresponds to the 120-cell's dodecahedron of type 3.  Make one.  The only polygons you need are of types already found in the snowman.

In fact, any structure which you can make out of just blue struts can be analogously squished in these three ways.  (All blue directions appear in the dodecahedron, so these are the only types struts you will need.)  Try making one of the three squished icosahedra (answer: see Exploration 2A), or one of the three squished truncated icosidodecahedra or one of the three squished stellated dodecahedra.

If you have tried Exploration 25Z, then you may understand the snowman as the projection of three cells of the 4D polytope that consists of 120 truncated icosahedra and 600 truncated tetrahedra.